CONDITION OF EQUILLIBRIUM

 

PRINCIPLE

A body or particle is said to be in equilibrium if the net resultant force acting on a body becomes zero i.e. the net resultant effect of the body is in stable condition.

EXPLANATION

 

R² = Σf_x²+ Σf_y²,  R = 0 , (for equilibrium condition)

Σf_x²+ Σf_y² = 0, Σf_x = 0, Σf_y = 0 Σm_A = 0

CONDITION

R² = Σf_x²+ Σf_y² (according to principle of equilibrium of force)

R = 0, Σf_x²+ Σf_y² = 0, Σf_x = 0, Σf_y = 0 Σm_A = 0

A body or particle is said to be in equilibrium if the following conditions are satisfied

1. Algebric sum of all the forces are acting in the X – direction is equal to zero [(  ͢+) Σf_x = 0] 
2. Algebric sum of all the forces are acting in the Y – direction is equal to zero [ (+↑)Σf_y = 0]
3. Algebric sum of all the moments of all the forces about any point or axis is equal to zero [ΣM_A = 0]

FREE BODY DIAGRAM(F.B.D)

for the shake of analysis we can draw the figure  seperatly in free space swoing all the active forces and the reactive forces replacing all the contact surface called the free body diagram / space diagram /F.B.D 

BOW’S NOTATION

DEFINATION

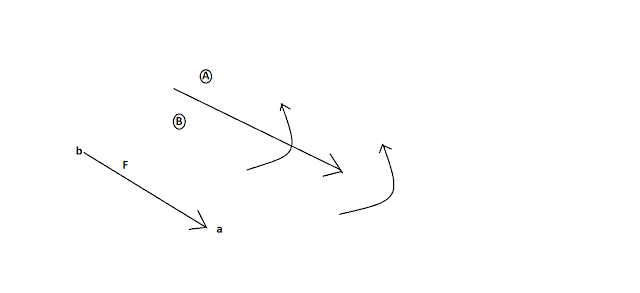
For graphical representation of a force same conventional methodology is adopted to denote the force , called bow’s notation.

In bow’s notation , two English capital latter is palsed on each side of the line of action of force

In figure force F is represented by (A) (B) bow’s notation.

EXAMPLE

let F₁, F₂ & F₃ are the forces are acting on a body and R be the resultant of all the forces as soon in figure.

(A)(B)(C)(D) are the notation are used to represent forces.

(A)(B) → F₁ & ab = F₁

(B)(C) → F₂ & bc = F₂

(C)(D) → F₃ & cd = F₃

(D)(A) → F₄ & da = F₄

[A represents the area between F₁ & R]

LAMI’S THEOREM

STATEMENT 

“If three coplanar forces are meeting at a point in rigid body or particle be the equilibrium then each force is directly proportional to the “sine” of the angle between the other two forces”

 EXPLANATION

Let , P, Q, R are three forces acting on a particle as shown in figure.According to the Lami’s theorem

P α sine α ; P = K sine α

Q α sine β ; Q = K sine β     [ K = constant]

R α sine λ ; R = K sine λ

Constant = K = P/ sine α  = Q/sine β = R/ sine λ

PROOF

From , ∆ABC

Now from triangle law we can say                                                                   

AB/sine(180ᶱ - λ) = BC/sine(180ᶱ - α) = AC/sine(180ᶱ - β)

P/ sine α  = Q/sine β = R/ sine λ (proof)